Decay and production of substances in the groundwater can be both of chemical and biological nature.
In the mass transport calculation decay or production processes can be taken into account. In theory, a distinction is made between production and decay rates of the solution in the fluid and the adsorbate in the matrix. In nature, these processes are not clearly separated from each other. Because of this, the mass transport calculation is done without taking the processes within the matrix into account. Instead, all production, degradation or decay processes are considered only in the fluid.
For the degradation and production in the fluid, different Ansatz functions are available:

No production and decay processes are considered (default).

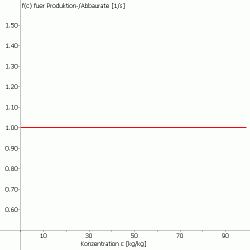
Constant behaviour of production and degradation rates:

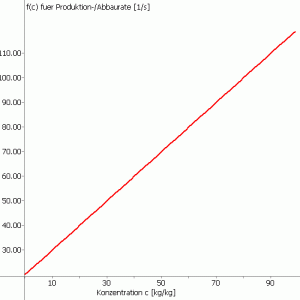
Linear behaviour of production and degradation rates:

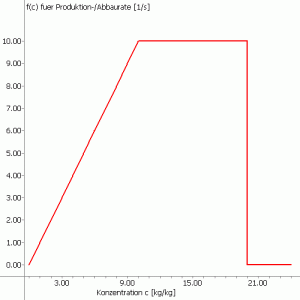
A step function can be entered for the decay and production rate (starting with f(c = 0) = 0), which is limited by a maximum rate (maximum) when reaching a certain concentration (lower limit). When reaching an entered a maximum concentration (upper limit) the decay or the production rate is set back to 0:


If a zoning of the material parameters was made (attribute Z-AP), a 4th Ansatz function for the decay and production rate is additionally available. The tent function drops after reaching a maximum until the upper limit is reached. After that the decay or the production rate is set back to 0:
In the example the lower limit is = 20.0, the upper limit = 30.0 and the maximum = 200.0.
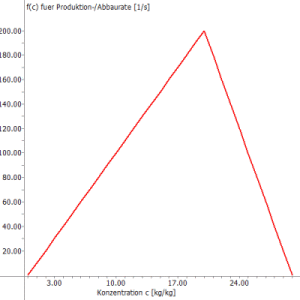
The individual parameters intercept, maximum, lower and upper limit of the function are defined in the extended definitions – decay and production .
 Radioactive or biological decay (half-life calculation)
Radioactive or biological decay (half-life calculation)
