Hubbert's Law is a relationship between the hydrostatic pressure p and potential head h:

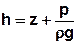
with:

h = potential head [m]

z = elevation [m]

p = pressure [N/m²] = [kg/(s²m)]

ρ = Density [kg/m³]

g = gravitational acceleration [m/s²]

 

One of the flowing medium independent parameter for the permeability K is the permeability K_perm. The relationship between the permeability K_perm of a porous medium and the permeability K of the porous medium in terms of a flowing fluid is:

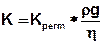
with:

 

K = symmetric tensor for the K_f-Wert [m/s]

K_perm = Permeability [m²]

ρ = Density [kg/m³]

g = gravitational acceleration [m/s²]

η = dynamic viscosity [kg/(ms)]

 

Inserting these two equations in the from the potential dependent steady state flow equation, we obtain the steady state flow equation as a function of pressure:

 

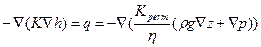
The unit of the equation is that of the source term q = [1/s].

Since unsaturated conditions are to be taken into account, the K_f-value has to be scaled with a relative k-value kr_el (0<k_rel<1).

 

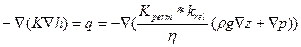
The factor k_rel [-] is defined as a function of the saturation: k_rel = k_rel (S_r). In the saturated zone is k_rel = 1.0.

The relative saturation is a variable, dependent of the pressure S_r = S_r(p), which is usually defined with a function according to van Genuchten.

 

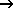Pressure-saturation function by van Genuchten