The non-linear relationship between capillary pressure and saturation is primarily determined by the nature of the soil (grain shape and grain size distribution). The following figure shows schematically the pressure-saturation relationship in the soil:

 

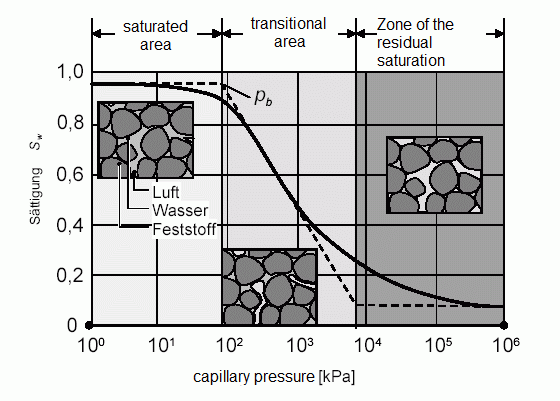
Capillary pressure-saturation relationship (pb means the water inflow pressure)

 

Van Genuchten defined the following relationship between the proportion of unsaturated from the saturated hydraulic conductivity:

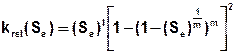
Meaning:

 

S_e = effective saturation

l = unknown parameter, determined by van Genuchten with the value 0,5

m = 1-1/n = constant, n = pore size index (> 1)

 

Van Genuchten [VAN GENUCHTEN, M. TH. (1980): A Closed-Form Equation for Predicting the Hydraulic Conductivity of Unsaturated Soils, Soil Science Society of Journal. 44: Pages 892-898. 1980] defined the values 1,5 (clayey) to 4,5 (sandy) for n.

 

The effective saturation S_e is defined by the following formula:

 

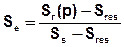
The parameters are:

 

S_r = relative saturation S_r(p)

S_res = Residual saturation = minimum saturation level, which exists as a result of flow processes and depends on the type of soil

S_s = maximum saturation = saturation level which is depends on the soil type and is at maximum attainable (S_s ~1.0)

 

The effective saturation is related to the capillary pressure p_c and the water inflow pressure p_e as follows:

 

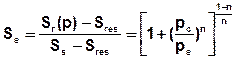
Solving this equation for the pressure-dependent relative saturation S_r(p), you obtained the pressure-saturation function according to van Genuchten on which the calculation in SPRING is based.

 

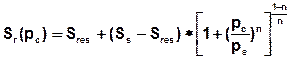
With:

p_c = capillary pressure [N/m²] = [kg/(s²m)]

p_e = water inflow pressure [N/m²] = [kg/(s²m)]

 

The water inflow pressure is a soil specific parameter. It is defined as the inverse of a = 1/p_e in the Saturation parameters. The following figure shows examples of the pressure-saturation functions according to van Genuchten depending on various soils:

 

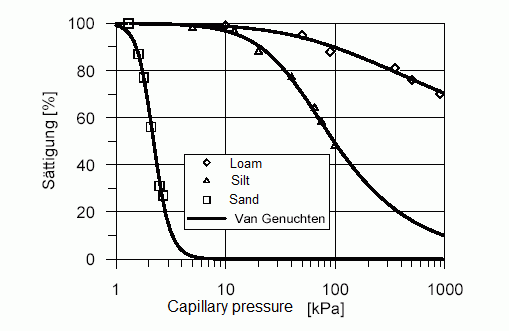
Pressure-saturation functions according to van Genuchten

 

Calculation of the free surface (2D)