In the flow field, a streamline represents a dividing line through which no water passes. Therefore, the mass flow rate of water between two streamlines is constant (assuming no infiltration from the top). These theoretical basics limit the calculation of streamlines related to mass flow rates in 2D horizontal models.

An important aspect for streamlines, which is not taken into account for path lines, is the transition between two elements with different thicknesses. Mass balance requires closer streamlines in larger thicknesses than it does in smaller thicknesses. The streamline results from an equation system at each node. The mass balance defines that the inflow rate must always be equal to the outflow rate (plus possible infiltration rates).

The starting points are also determined by the mass balance for the nodes. First, a limit mass rate per streamline must be defined. The number of streamlines at each well node results from dividing the mass flow rates at the nodes by the limit rate. The streamlines are distributed to the adjacent element sides depending on the mass flow rates passing them. Starting at an arbitrary point, the rates are summed until the sum reaches the limit rate. At this point, the next streamline has its starting point. Starting at the computed points, the streamlines are followed through the next element and again over a side, and so on. In the same way, starting points along the outer boundary or receiving water courses are determined. The inflow and outflow rates are summed until the limit is reached.

The backwards-computed streamlines end at the side of an element adjacent to a node which has an inflow. This can be either a boundary node with a computed inflow rate or any node of the model with an infiltration rate (even from areal recharge).

In general, a horizontal model must take into account the areal recharge rate. It is assigned to all nodes as a small infiltration rate. Therefore, each node can be the endpoint of a backwards-computed streamline. This results in less fewer lines, e.g. along the drainage area of an exfiltration well, and represents the distribution of areal recharge. However, this effect does become apparent until a large number of streamlines per well has been generated.

The computed streamlines not only show the flow paths of the groundwater, but give an overview of the flow rates. Very close streamlines represent large mass flow rates, while widely separated ones represent small mass flow rates. In addition, the quality of the calibration can be checked with the help of streamlines. Individual elements with a much higher or lower permeability than the adjacent elements cause sharp bends of the stream lines.

The graphic representation of streamlines strongly depends on the quality of the finite element mesh, because large discontinuities at the element boundaries cause lateral displacements of the lines.

 

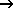Calculating and plotting stream lines