The hydromechanical dispersion describes the distribution and the mixing of the same materials (or heat) in the moving pore water. This process is due to the different flow rates in the pore, the pore size distribution and the path which each particle covers.

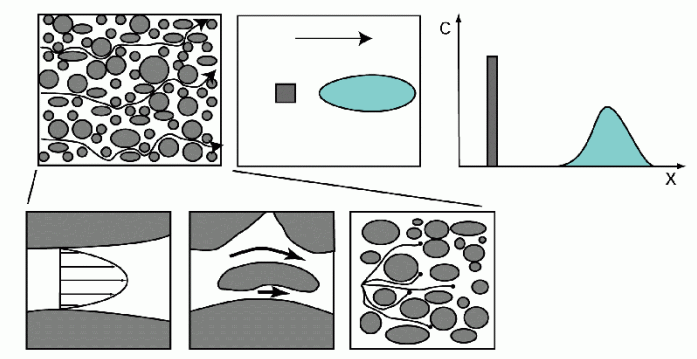
Dispersive mass transport

The hydromechanical dispersion provides a further flow-dependent component to the mass or energy flow besides the advective component. The mass or energy flow is described with the 1st Fick's law, similar to the diffusion:

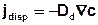
With the symmetrical dispersion tensor D_d [m²/s]:

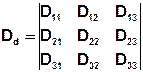
Its coefficients in the three-dimensional analysis are defined as follows by Frind (from: FRIND, E.O.; BURNETT, R. D. (1987): Simulation of contaminant transport in three dimensions, 2. Dimensionality effects. Water Resour. Res., 23(4), pp. 695-705):

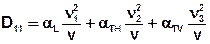
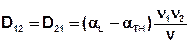
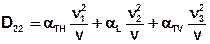
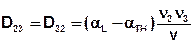
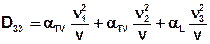
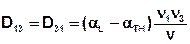
with:

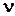= (v₁, v₂, v₃) = velocities in the carthesian coordinate system [m/s],

In the two-dimensional, the coefficients of the dispersion tensor D_d are defined by Scheidegger:

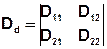
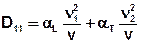
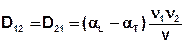
with:

α_T = transversal dispersivity [m],

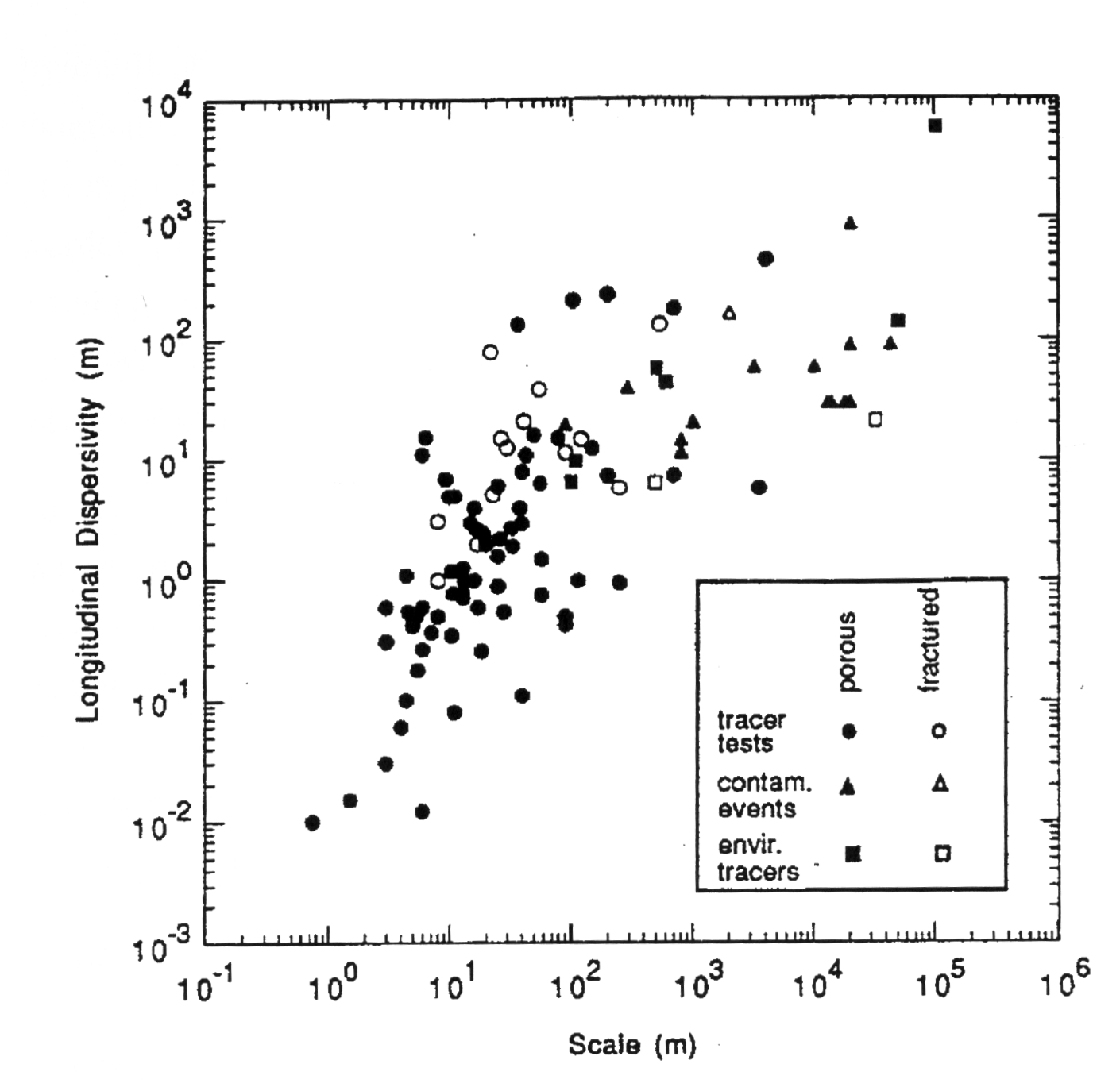
The dispersivity is a characteristic length that can be measured in the laboratory or in the field. It is a geometric measure of the permeability and storage heterogeneity of the aquifer. The magnitude of the dispersion coefficient α is dependent on the scale as well as of the porosity, grain shape and grain size.

The hydrodynamic (total) dispersion results from the addition of the hydromechanical dispersion and the diffusion.

Substances which obey only the previously described so-called conservative transport processes are known as an ideal tracer.

In the following the so-called non-conservative mechanisms are described which cause a retention (retardation) and a reduction in the mass content in the groundwater.

Adsorption