The differential equations described above are supplemented by the boundary conditions:
Boundary condition 1st order: Specification of fixed concentrations (attribute 1KON):
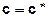
Boundary condition 2nd order: Specification of a diffusive-dispersive mass flux on the boundary. The almost exclusive practical application, however, is to make the edge impermeable for diffusion and dispersion, so that:
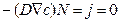
(N denotes the outward facing normal to the boundary of the model).
The system of equations can only be solved if at least at one node a boundary condition of the 1st order is defined.
For a transient mass transport equation initial conditions for the start time have to be defined for the entire model domain.
Initial condition: Initial concentrations for the start time t0 of the transient calculation (attribute AKON):
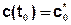
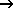Input parameters